Thomas Spencer (mathematical physicist)
Thomas C. Spencer (born December 24, 1946) is an American mathematical physicist, known in particular for important contributions to constructive quantum field theory, statistical mechanics, and spectral theory of random operators.[1] Since 1986, he is professor of mathematics at the Institute for Advanced Study. He is a member of the United States National Academy of Sciences,[1] and the recipient of the Dannie Heineman Prize for Mathematical Physics (joint with Jürg Fröhlich, "For their joint work in providing rigorous mathematical solutions to some outstanding problems in statistical mechanics and field theory.").[2][3]
Main Results
- Together with James Glimm and Arthur Jaffe he invented the cluster expansion approach to quantum field theory that is widely used in constructive field theory also nowadays.[4]
- Together with Jürg Fröhlich and Barry Simon, he invented the approach of the infrared bound, nowadays become a classical tool to show phase transitions in various models of statistical mechanics.[5]
- Together with Jürg Fröhlich, he devised a 'multi-scale analysis' to provide, for the first time, mathematical proofs of: the Kosterlitz–Thouless transition;[6] the phase transition in the one-dimensional ferromagnetic Ising model with interactions
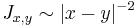;[7] the Anderson localization in arbitrary dimension.[8]
- Together with David Brydges, he proved that the scaling limit of the self-avoiding walk in dimension greater or equal than 5 is Gaussian, with variance growing linearly in time.[9] To achieve this result, they invented the technique of the lace expansion that since then has had wide application in probability on graphs.[10]
References
- ^ a b IAS website
- ^ APS website
- ^ 1991 Dannie Heineman Prize for Mathematical Physics Recipient, American Physical Society. Accessed June 24, 2011
- ^ Glimm, J; Jaffe, A; Spencer, T (1974). "The Wightman axioms and particle structure in the
quantum field model". Ann.of Math 100: 585–632. JSTOR 1970959. - ^ Fröhlich, J.; Simon, B.; Spencer, T. (1976). "Infrared bounds, phase transitions and continuous symmetry breaking". Comm. Math. Phys. 50 (1): 79–95. http://www.springerlink.com/content/p000130r85020560/.
- ^ Fröhlich, J.; Spencer, T. (1981). "The Kosterlitz–Thouless transition in two-dimensional abelian spin systems and the Coulomb gas". Comm. Math. Phys. 81 (4): 527–602. http://projecteuclid.org/euclid.cmp/1103920388.
- ^ Fröhlich, J.; Spencer, T. (1982). "The phase transition in the one-dimensional Ising model with 1/r2 interaction energy.". Comm. Math. Phys. 84 (1): 87–101. http://www.springerlink.com/content/wu3782848714tt0l.
- ^ Fröhlich, J.; Spencer, T. (1983). "Absence of diffusion in the Anderson tight binding model for large disorder or low energy.". Comm. Math. Phys. 88 (2): 151–184. http://projecteuclid.org/getRecord?id=euclid.cmp/1103922279.
- ^ Brydges, D.; Spencer, T. (1985). "Self-avoiding walk in 5 or more dimensions". Comm. Math. Phys. 97 (1–2): 125–148. http://projecteuclid.org/euclid.cmp/1103941982.
- ^ Slade, G. (2006). "The lace expansion and its applications".Lecture Notes in Mathematics. 1879. Springer. http://www.springer.com/mathematics/probability/book/978-3-540-31189-8.